How our GPS coordinate is determined: Trilateration
Posted: Fri Jan 30, 2009 10:34 pm
Trilateration
Selected and re-edited by FauSham from many resources as Informative sources for MFM Reader.
Did u know that, our GPS use Trilateration geometric equation to calculate our coordinate?
But I like to share with all MFM's member.....
What is Trilateration?
The measurement of a series of distances between points on the surface of the earth, for the purpose of establishing relative positions of the points in surveying.
Trilateration is a method for determining the intersections of three sphere surfaces given the centers and radii of the three spheres. huerm....... my gps device so brilliant... math-power.....
huerm....... my gps device so brilliant... math-power.....
See this diagram.....

We start with the equations for the three spheres


and

We need to find a point located at (x, y, z) that satisfies all three equations.
First we subtract the second equation from the first and solve for x:

Substituting this back into the formula for the first sphere produces the formula for a circle, the solution to the intersection of the first two spheres:

Substituting : into the formula for the third sphere and solving for y there results:
into the formula for the third sphere and solving for y there results:

Now that we have the x- and y-coordinates of the solution point, we can simply rearrange the formula for the first sphere to find the z-coordinate:

All this calculation has been made and use by our GPS to do tracking
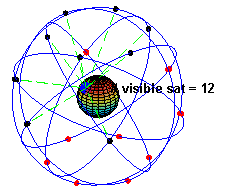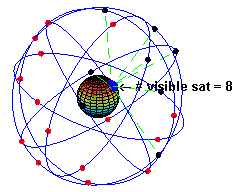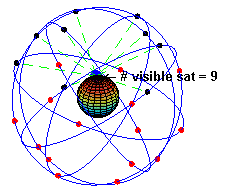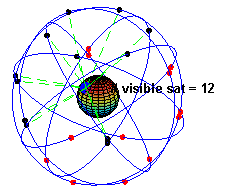
Diagram credit: Wikipedia
Selected and re-edited by FauSham from many resources as Informative sources for MFM Reader.
Did u know that, our GPS use Trilateration geometric equation to calculate our coordinate?
But I like to share with all MFM's member.....
What is Trilateration?
The measurement of a series of distances between points on the surface of the earth, for the purpose of establishing relative positions of the points in surveying.
Trilateration is a method for determining the intersections of three sphere surfaces given the centers and radii of the three spheres.
 huerm....... my gps device so brilliant... math-power.....
huerm....... my gps device so brilliant... math-power.....See this diagram.....

We start with the equations for the three spheres


and

We need to find a point located at (x, y, z) that satisfies all three equations.
First we subtract the second equation from the first and solve for x:

Substituting this back into the formula for the first sphere produces the formula for a circle, the solution to the intersection of the first two spheres:

Substituting :
 into the formula for the third sphere and solving for y there results:
into the formula for the third sphere and solving for y there results:
Now that we have the x- and y-coordinates of the solution point, we can simply rearrange the formula for the first sphere to find the z-coordinate:

All this calculation has been made and use by our GPS to do tracking


Diagram credit: Wikipedia